

Relation and Functions Worksheet (pdf) with Key
Students will practice classifying relations ( functions vs relations ) from graphs, equations and ordered pairs. Also, students will identify the domain and range of a given relation/function.
Example Questions
Directions : Determine if the relation is a function .

Directions : State the domain of each relation/function .

Challenge Problems

- Relations and Functions - everything you might want to know!
- Domain and Range
- Functions and Relations in Math
Ultimate Math Solver (Free) Free Algebra Solver ... type anything in there!
Popular pages @ mathwarehouse.com.

- Rating Count
- Price (Ascending)
- Price (Descending)
- Most Recent
Graphs of real world situations
Resource type.
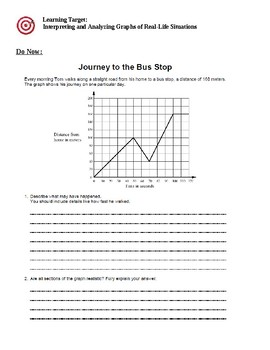
Interpreting and Analyzing Graphs of Real-Life Situations

Properties of Functions Unit | Identify, Evaluate, & Graph Functions

Writing Solving Graphing One Step Inequalities 6th Grade Practice Activities

Graphing and Writing Equations of Circles: Cell Phone Towers Task

Systems of Linear Equations & Inequalities - Word Problems for Google Slides™

Systems of Equations and Inequalities - Real World Applications

Writing Equations of Lines - Real World Linear Functions Project Algebra 1

Task Cards: Calculate Rate of Change in Real - World Situations

Scavenger Hunt Activity: Analyze Graphs of Real World Linear Functions
Solving Systems of Linear Equations by Graphing Google Forms Homework

Creating a Graph from a Table of Values Worksheet

Interpreting Graphs Unit Bundle of Activities

Slope from Real World Graphs Activity Scavenger Hunt Digital and Printable

Real - World Problems using Linear Functions Worksheet

Solutions to Graphed Linear Systems of Equations Differentiated Worksheets
Rate of Change ( Graphs and Tables) and Functions Practice Worksheet

Real World Graphing | Digital Math Activities

Solving Systems of Equations by Graphing Worksheet

System of Linear Equations Digital Bundle: Graphing , Elimination, Substitution

Finding Slope, Graphing Lines, and Writing Equations Notes Bundle - 11 Lessons!!

Comparing Linear, Quadratic, and Exponential Graphs Google Slides Activity

Functions & Equations Linear Nonlinear Rate of Change Tables & Graphs & Systems

Graph Quadratic Functions from Factored Form NO PREP Student-Led Lesson

Graphing and Writing Systems of Linear Inequalities Sketch Notes & Activity

- We're hiring
- Help & FAQ
- Privacy policy
- Student privacy
- Terms of service
- Tell us what you think
- Kindergarten
- Greater Than Less Than
- Measurement
- Multiplication
- Place Value
- Subtraction
- Punctuation
- 1st Grade Reading
- 2nd Grade Reading
- 3rd Grade Reading
- Cursive Writing
- Alphabet Coloring
- Animals Coloring
- Birthday Coloring
- Boys Coloring
- Buildings Coloring
- Cartoons Coloring
- Christmas Coloring
- Country Flag Coloring
- Country Map Coloring
- Disney Coloring
- Fantasy Coloring
- Food Coloring
- Girls Coloring
- Holidays Coloring
- Music Coloring
- Nature Coloring
- New Year Coloring
- People Coloring
- Religious Coloring
- Sports Coloring
- Toys Coloring
- Transportation Coloring
- US Sports Team Coloring
- Valentine Day Coloring
Homework 2 Real World Graphs
Displaying top 8 worksheets found for - Homework 2 Real World Graphs .
Some of the worksheets for this concept are Systems of equations real world graphing, Real world graphs, Real life graphs travel conversion, Name date ms, Real world linear equation work and activity, Unit 2 2 writing and graphing quadratics work practice packet, Solving real life problems, Real world linear equation work and activity.
Found worksheet you are looking for? To download/print, click on pop-out icon or print icon to worksheet to print or download. Worksheet will open in a new window. You can & download or print using the browser document reader options.
1. Systems of equations Real World graphing
2. 1.1 real-world graphs, 3. real life graphs (travel, conversion), 4. name date ms, 5. real world linear equation worksheet and activity, 6. unit 2-2: writing and graphing quadratics worksheet practice packet, 7. 3.4 solving real-life problems, 8. real world linear equation worksheet and activity.
MTH 111 (Online) Walkthrough Text
Walkthrough of unit 3: rates of change and behavior of graphs and composition of functions, learning outcomes.
- Find the average rate of change of a function.
- Use a graph to determine where a function is increasing, decreasing, or constant.
- Use a graph to locate local and absolute maxima and local minima.
- Combine functions using algebraic operations.
- Create a new function by composition of functions.
- Evaluate composite functions.
- Find the domain of a composite function.
- Decompose a composite function into its component functions.
Rates of Change
Gasoline costs have experienced some wild fluctuations over the last several decades. The table below [1] lists the average cost, in dollars, of a gallon of gasoline for the years 2005–2012. The cost of gasoline can be considered as a function of year.
| 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | |
| 2.31 | 2.62 | 2.84 | 3.30 | 2.41 | 2.84 | 3.58 | 3.68 |
If we were interested only in how the gasoline prices changed between 2005 and 2012, we could compute that the cost per gallon had increased from $2.31 to $3.68, an increase of $1.37. While this is interesting, it might be more useful to look at how much the price changed per year . In this section, we will investigate changes such as these.
Finding the Average Rate of Change of a Function
The price change per year is a rate of change because it describes how an output quantity changes relative to the change in the input quantity. We can see that the price of gasoline in the table above did not change by the same amount each year, so the rate of change was not constant. If we use only the beginning and ending data, we would be finding the average rate of change over the specified period of time. To find the average rate of change, we divide the change in the output value by the change in the input value.
The Greek letter [latex]\Delta [/latex] (delta) signifies the change in a quantity; we read the ratio as “delta- y over delta- x ” or “the change in [latex]y[/latex] divided by the change in [latex]x[/latex].” Occasionally we write [latex]\Delta f[/latex] instead of [latex]\Delta y[/latex], which still represents the change in the function’s output value resulting from a change to its input value. It does not mean we are changing the function into some other function.
In our example, the gasoline price increased by $1.37 from 2005 to 2012. Over 7 years, the average rate of change was
On average, the price of gas increased by about 19.6¢ each year.
Other examples of rates of change include:
- A population of rats increasing by 40 rats per week
- A car traveling 68 miles per hour (distance traveled changes by 68 miles each hour as time passes)
- A car driving 27 miles per gallon (distance traveled changes by 27 miles for each gallon)
- The current through an electrical circuit increasing by 0.125 amperes for every volt of increased voltage
- The amount of money in a college account decreasing by $4,000 per quarter
A General Note: Rate of Change
A rate of change describes how an output quantity changes relative to the change in the input quantity. The units on a rate of change are “output units per input units.”
The average rate of change between two input values is the total change of the function values (output values) divided by the change in the input values.
How To: Given the value of a function at different points, calculate the average rate of change of a function for the interval between two values [latex]{x}_{1}[/latex] and [latex]{x}_{2}[/latex].
- Calculate the difference [latex]{y}_{2}-{y}_{1}=\Delta y[/latex].
- Calculate the difference [latex]{x}_{2}-{x}_{1}=\Delta x[/latex].
- Find the ratio [latex]\frac{\Delta y}{\Delta x}[/latex].
Example 1: Computing an Average Rate of Change
Using the data in the table below, find the average rate of change of the price of gasoline between 2007 and 2009.
In 2007, the price of gasoline was $2.84. In 2009, the cost was $2.41. The average rate of change is
[latex]\begin{align}\frac{\Delta y}{\Delta x}&=\frac{{y}_{2}-{y}_{1}}{{x}_{2}-{x}_{1}} \\[1mm] &=\frac{2.41-2.84}{2009 - 2007} \\[1mm] &=\frac{-0.43}{2\text{ years}} \\[1mm] &={-0.22}\text{ per year}\end{align}[/latex]
Analysis of the Solution
Note that a decrease is expressed by a negative change or “negative increase.” A rate of change is negative when the output decreases as the input increases or when the output increases as the input decreases.
The following video provides another example of how to find the average rate of change between two points from a table of values.
Using the data in the table below, find the average rate of change between 2005 and 2010.
| 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | |
| 2.31 | 2.62 | 2.84 | 3.30 | 2.41 | 2.84 | 3.58 | 3.68 |
[latex]\dfrac{$2.84-$2.31}{5\text{ years}}=\dfrac{$0.53}{5\text{ years}}=$0.106[/latex] per year.

Example 2: Computing Average Rate of Change from a Graph
Given the function [latex]g\left(t\right)[/latex] shown in Figure 1, find the average rate of change on the interval [latex]\left[-1,2\right][/latex].

At [latex]t=-1[/latex], the graph shows [latex]g\left(-1\right)=4[/latex]. At [latex]t=2[/latex], the graph shows [latex]g\left(2\right)=1[/latex].
The horizontal change [latex]\Delta t=3[/latex] is shown by the red arrow, and the vertical change [latex]\Delta g\left(t\right)=-3[/latex] is shown by the turquoise arrow. The output changes by –3 while the input changes by 3, giving an average rate of change of
[latex]\frac{1 - 4}{2-\left(-1\right)}=\frac{-3}{3}=-1[/latex]
Note that the order we choose is very important. If, for example, we use [latex]\frac{{y}_{2}-{y}_{1}}{{x}_{1}-{x}_{2}}[/latex], we will not get the correct answer. Decide which point will be 1 and which point will be 2, and keep the coordinates fixed as [latex]\left({x}_{1},{y}_{1}\right)[/latex] and [latex]\left({x}_{2},{y}_{2}\right)[/latex].
Example 3: Computing Average Rate of Change from a Table
After picking up a friend who lives 10 miles away, Anna records her distance from home over time. The values are shown in the table below. Find her average speed over the first 6 hours.
| (hours) | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| ( ) (miles) | 10 | 55 | 90 | 153 | 214 | 240 | 292 | 300 |
Here, the average speed is the average rate of change. She traveled 282 miles in 6 hours, for an average speed of
[latex]\frac{292 - 10}{6 - 0} =\frac{282}{6} =47[/latex]
The average speed is 47 miles per hour.
Because the speed is not constant, the average speed depends on the interval chosen. For the interval [2,3], the average speed is 63 miles per hour.
Example 4: Computing Average Rate of Change for a Function Expressed as a Formula
Compute the average rate of change of [latex]f\left(x\right)={x}^{2}-\frac{1}{x}[/latex] on the interval [latex]\text{[2,}\text{4].}[/latex]
We can start by computing the function values at each endpoint of the interval.
[latex]\begin{align}f\left(2\right)&={2}^{2}-\frac{1}{2} &&& f\left(4\right)&={4}^{2}-\frac{1}{4} \\ &=4-\frac{1}{2} &&&& =16-\frac{1}{4} \\ &=\frac{7}{2} &&&& =\frac{63}{4} \end{align}[/latex]
Now we compute the average rate of change.
[latex]\begin{align}\text{Average rate of change}&=\frac{f\left(4\right)-f\left(2\right)}{4 - 2} \\[1mm] &=\frac{\frac{63}{4}-\frac{7}{2}}{4 - 2} \\[1mm] &=\frac{\frac{49}{4}}{2} \\[1mm] &=\frac{49}{8} \end{align}[/latex]
The following video provides another example of finding the average rate of change of a function given a formula and an interval.
Find the average rate of change of [latex]f\left(x\right)=x - 2\sqrt{x}[/latex] on the interval [latex]\left[1,9\right][/latex].
[latex]\frac{1}{2}[/latex]
Example 5: Finding the Average Rate of Change of a Force
The electrostatic force [latex]F[/latex], measured in newtons, between two charged particles can be related to the distance between the particles [latex]d[/latex], in centimeters, by the formula [latex]F\left(d\right)=\frac{2}{{d}^{2}}[/latex]. Find the average rate of change of force if the distance between the particles is increased from 2 cm to 6 cm.
We are computing the average rate of change of [latex]F\left(d\right)=\frac{2}{{d}^{2}}[/latex] on the interval [latex]\left[2,6\right][/latex].
[latex]\begin{align}\text{Average rate of change }&=\frac{F\left(6\right)-F\left(2\right)}{6 - 2} \\[1mm] &=\frac{\frac{2}{{6}^{2}}-\frac{2}{{2}^{2}}}{6 - 2} && \text{Simplify}. \\[1mm] &=\frac{\frac{2}{36}-\frac{2}{4}}{4} \\[1mm] &=\frac{-\frac{16}{36}}{4} &&\text{Combine numerator terms}. \\[1mm] &=-\frac{1}{9}&&\text{Simplify}\end{align}[/latex]
The average rate of change is [latex]-\frac{1}{9}[/latex] newton per centimeter.
Example 6: Finding an Average Rate of Change as an Expression
Find the average rate of change of [latex]g\left(t\right)={t}^{2}+3t+1[/latex] on the interval [latex]\left[0,a\right][/latex]. The answer will be an expression involving [latex]a[/latex].
We use the average rate of change formula.
[latex]\begin{align}\text{Average rate of change}&=\frac{g\left(a\right)-g\left(0\right)}{a - 0}&&\text{Evaluate} \\[1mm] &=\frac{\left({a}^{2}+3a+1\right)-\left({0}^{2}+3\left(0\right)+1\right)}{a - 0}&&\text{Simplify} \\[1mm] &=\frac{{a}^{2}+3a+1 - 1}{a}&&\text{Simplify and factor} \\[1mm] &=\frac{a\left(a+3\right)}{a}&&\text{Divide by the common factor }a \\[1mm] &=a+3 \end{align}[/latex]
This result tells us the average rate of change in terms of [latex]a[/latex] between [latex]t=0[/latex] and any other point [latex]t=a[/latex]. For example, on the interval [latex]\left[0,5\right][/latex], the average rate of change would be [latex]5+3=8[/latex].
Find the average rate of change of [latex]f\left(x\right)={x}^{2}+2x - 8[/latex] on the interval [latex]\left[5,a\right][/latex].
[latex]a+7[/latex]
As part of exploring how functions change, we can identify intervals over which the function is changing in specific ways. We say that a function is increasing on an interval if the function values increase as the input values increase within that interval. Similarly, a function is decreasing on an interval if the function values decrease as the input values increase over that interval. The average rate of change of an increasing function is positive, and the average rate of change of a decreasing function is negative. Figure 3 shows examples of increasing and decreasing intervals on a function.

Figure 3. The function [latex]f\left(x\right)={x}^{3}-12x[/latex] is increasing on [latex]\left(-\infty \text{,}-\text{2}\right){{\cup }^{\text{ }}}^{\text{ }}\left(2,\infty \right)[/latex] and is decreasing on [latex]\left(-2\text{,}2\right)[/latex].
This video further explains how to find where a function is increasing or decreasing.
While some functions are increasing (or decreasing) over their entire domain, many others are not. A value of the input where a function changes from increasing to decreasing (as we go from left to right, that is, as the input variable increases) is called a local maximum . If a function has more than one, we say it has local maxima. Similarly, a value of the input where a function changes from decreasing to increasing as the input variable increases is called a local minimum . The plural form is “local minima.” Together, local maxima and minima are called local extrema , or local extreme values, of the function. (The singular form is “extremum.”) Often, the term local is replaced by the term relative . In this text, we will use the term local .
Clearly, a function is neither increasing nor decreasing on an interval where it is constant. A function is also neither increasing nor decreasing at extrema. Note that we have to speak of local extrema, because any given local extremum as defined here is not necessarily the highest maximum or lowest minimum in the function’s entire domain.
For the function in Figure 4, the local maximum is 16, and it occurs at [latex]x=-2[/latex]. The local minimum is [latex]-16[/latex] and it occurs at [latex]x=2[/latex].

To locate the local maxima and minima from a graph, we need to observe the graph to determine where the graph attains its highest and lowest points, respectively, within an open interval. Like the summit of a roller coaster, the graph of a function is higher at a local maximum than at nearby points on both sides. The graph will also be lower at a local minimum than at neighboring points. Figure 5 illustrates these ideas for a local maximum.

Figure 5. Definition of a local maximum.
These observations lead us to a formal definition of local extrema.
A General Note: Local Minima and Local Maxima
A function [latex]f[/latex] is an increasing function on an open interval if [latex]f\left(b\right)>f\left(a\right)[/latex] for any two input values [latex]a[/latex] and [latex]b[/latex] in the given interval where [latex]b>a[/latex].
A function [latex]f[/latex] is a decreasing function on an open interval if [latex]f\left(b\right)<f\left(a\right)[/latex] for any two input values [latex]a[/latex] and [latex]b[/latex] in the given interval where [latex]b>a[/latex].
A function [latex]f[/latex] has a local maximum at [latex]x=b[/latex] if there exists an interval [latex]\left(a,c\right)[/latex] with [latex]a<b<c[/latex] such that, for any [latex]x[/latex] in the interval [latex]\left(a,c\right)[/latex], [latex]f\left(x\right)\le f\left(b\right)[/latex]. Likewise, [latex]f[/latex] has a local minimum at [latex]x=b[/latex] if there exists an interval [latex]\left(a,c\right)[/latex] with [latex]a<b<c[/latex] such that, for any [latex]x[/latex] in the interval [latex]\left(a,c\right)[/latex], [latex]f\left(x\right)\ge f\left(b\right)[/latex].
Example 7: Finding Increasing and Decreasing Intervals on a Graph
Given the function [latex]p\left(t\right)[/latex] in the graph below, identify the intervals on which the function appears to be increasing.

We see that the function is not constant on any interval. The function is increasing where it slants upward as we move to the right and decreasing where it slants downward as we move to the right. The function appears to be increasing from [latex]t=1[/latex] to [latex]t=3[/latex] and from [latex]t=4[/latex] on.
In interval notation , we would say the function appears to be increasing on the interval (1,3) and the interval [latex]\left(4,\infty \right)[/latex].
Notice in this example that we used open intervals (intervals that do not include the endpoints), because the function is neither increasing nor decreasing at [latex]t=1[/latex] , [latex]t=3[/latex] , and [latex]t=4[/latex] . These points are the local extrema (two minima and a maximum).
Example 8: Finding Local Extrema from a Graph
Graph the function [latex]f\left(x\right)=\frac{2}{x}+\frac{x}{3}[/latex]. Then use the graph to estimate the local extrema of the function and to determine the intervals on which the function is increasing.
Using technology, we find that the graph of the function looks like that in Figure 7. It appears there is a low point, or local minimum, between [latex]x=2[/latex] and [latex]x=3[/latex], and a mirror-image high point, or local maximum, somewhere between [latex]x=-3[/latex] and [latex]x=-2[/latex].

Most graphing calculators and graphing utilities can estimate the location of maxima and minima. Figure 7 provides screen images from two different technologies, showing the estimate for the local maximum and minimum.

Based on these estimates, the function is increasing on the interval [latex]\left(-\infty ,-{2.449}\right)[/latex] and [latex]\left(2.449\text{,}\infty \right)[/latex]. Notice that, while we expect the extrema to be symmetric, the two different technologies agree only up to four decimals due to the differing approximation algorithms used by each. (The exact location of the extrema is at [latex]\pm \sqrt{6}[/latex], but determining this requires calculus.)
Graph the function [latex]f\left(x\right)={x}^{3}-6{x}^{2}-15x+20[/latex] to estimate the local extrema of the function. Use these to determine the intervals on which the function is increasing and decreasing.
The local maximum is 28 at x = -1 and the local minimum is -80 at x = 5. The function is increasing on [latex]\left(-\infty ,-1\right)\cup \left(5,\infty \right)[/latex] and decreasing on [latex]\left(-1,5\right)[/latex].

Example 9: Finding Local Maxima and Minima from a Graph
For the function [latex]f[/latex] whose graph is shown in Figure 9, find all local maxima and minima.

Observe the graph of [latex]f[/latex]. The graph attains a local maximum at [latex]x=1[/latex] because it is the highest point in an open interval around [latex]x=1[/latex]. The local maximum is the [latex]y[/latex] -coordinate at [latex]x=1[/latex], which is [latex]2[/latex].
The graph attains a local minimum at [latex]\text{ }x=-1\text{ }[/latex] because it is the lowest point in an open interval around [latex]x=-1[/latex]. The local minimum is the y -coordinate at [latex]x=-1[/latex], which is [latex]-2[/latex].
Analyzing the Toolkit Functions for Increasing or Decreasing Intervals
We will now return to our toolkit functions and discuss their graphical behavior in the table below.
| Function | Increasing/Decreasing | Example |
|---|---|---|
| Constant Function [latex]f\left(x\right)={c}[/latex] | Neither increasing nor decreasing | |
| Identity Function [latex]f\left(x\right)={x}[/latex] | Increasing | |
| Quadratic Function [latex]f\left(x\right)={x}^{2}[/latex] | Increasing on [latex]\left(0,\infty\right)[/latex] Decreasing on [latex]\left(-\infty,0\right)[/latex] Minimum at [latex]x=0[/latex] | |
| Cubic Function [latex]f\left(x\right)={x}^{3}[/latex] | Increasing | |
| Reciprocal [latex]f\left(x\right)=\frac{1}{x}[/latex] | Decreasing [latex]\left(-\infty,0\right)\cup\left(0,\infty\right)[/latex] | |
| Reciprocal Squared [latex]f\left(x\right)=\frac{1}{{x}^{2}}[/latex] | Increasing on [latex]\left(-\infty,0\right)[/latex] Decreasing on [latex]\left(0,\infty\right)[/latex] | |
| Cube Root [latex]f\left(x\right)=\sqrt[3]{x}[/latex]
| Increasing | |
| Square Root [latex]f\left(x\right)=\sqrt{x}[/latex] | Increasing on [latex]\left(0,\infty\right)[/latex] | |
| Absolute Value [latex]f\left(x\right)=|x|[/latex] | Increasing on [latex]\left(0,\infty\right)[/latex] Decreasing on [latex]\left(-\infty,0\right)[/latex] |
Composition of Functions
Suppose we want to calculate how much it costs to heat a house on a particular day of the year. The cost to heat a house will depend on the average daily temperature, and in turn, the average daily temperature depends on the particular day of the year. Notice how we have just defined two relationships: The cost depends on the temperature, and the temperature depends on the day.

Using descriptive variables, we can notate these two functions. The function [latex]C\left(T\right)[/latex] gives the cost [latex]C[/latex] of heating a house for a given average daily temperature in [latex]T[/latex] degrees Celsius. The function [latex]T\left(d\right)[/latex] gives the average daily temperature on day [latex]d[/latex] of the year. For any given day, [latex]\text{Cost}=C\left(T\left(d\right)\right)[/latex] means that the cost depends on the temperature, which in turns depends on the day of the year. Thus, we can evaluate the cost function at the temperature [latex]T\left(d\right)[/latex]. For example, we could evaluate [latex]T\left(5\right)[/latex] to determine the average daily temperature on the 5th day of the year. Then, we could evaluate the cost function at that temperature. We would write [latex]C\left(T\left(5\right)\right)[/latex].
By combining these two relationships into one function, we have performed function composition, which is the focus of this section.
Combining Functions Using Algebraic Operations
Function composition is only one way to combine existing functions. Another way is to carry out the usual algebraic operations on functions, such as addition, subtraction, multiplication and division. We do this by performing the operations with the function outputs, defining the result as the output of our new function.
Suppose we need to add two columns of numbers that represent a husband and wife’s separate annual incomes over a period of years, with the result being their total household income. We want to do this for every year, adding only that year’s incomes and then collecting all the data in a new column. If [latex]w\left(y\right)[/latex] is the wife’s income and [latex]h\left(y\right)[/latex] is the husband’s income in year [latex]y[/latex], and we want [latex]T[/latex] to represent the total income, then we can define a new function.
If this holds true for every year, then we can focus on the relation between the functions without reference to a year and write
Just as for this sum of two functions, we can define difference, product, and ratio functions for any pair of functions that have the same kinds of inputs (not necessarily numbers) and also the same kinds of outputs (which do have to be numbers so that the usual operations of algebra can apply to them, and which also must have the same units or no units when we add and subtract). In this way, we can think of adding, subtracting, multiplying, and dividing functions.
For two functions [latex]f\left(x\right)[/latex] and [latex]g\left(x\right)[/latex] with real number outputs, we define new functions [latex]f+g,f-g,fg[/latex], and [latex]\frac{f}{g}[/latex] by the relations
Example 1: Performing Algebraic Operations on Functions
Find and simplify the functions [latex]\left(g-f\right)\left(x\right)[/latex] and [latex]\left(\frac{g}{f}\right)\left(x\right)[/latex], given [latex]f\left(x\right)=x - 1[/latex] and [latex]g\left(x\right)={x}^{2}-1[/latex]. Are they the same function?
Begin by writing the general form, and then substitute the given functions.
[latex]\begin{align}\left(g-f\right)\left(x\right)&=g\left(x\right)-f\left(x\right) \\[1.5mm] &={x}^{2}-1-\left(x - 1\right)\\[1.5mm] &={x}^{2}-x \\[1.5mm] &=x\left(x - 1\right) \end{align}[/latex] [latex]\begin{align} \left(\frac{g}{f}\right)\left(x\right)&=\frac{g\left(x\right)}{f\left(x\right)} \\[1.5mm] &=\frac{{x}^{2}-1}{x - 1}\\[1.5mm] &=\frac{\left(x+1\right)\left(x - 1\right)}{x - 1}\text{ where }x\ne 1 \\[1.5mm] &=x+1 \end{align}[/latex]
No, the functions are not the same.
Note: For [latex]\left(\frac{g}{f}\right)\left(x\right)[/latex], the condition [latex]x\ne 1[/latex] is necessary because when [latex]x=1[/latex], the denominator is equal to 0, which makes the function undefined.
Find and simplify the functions [latex]\left(fg\right)\left(x\right)[/latex] and [latex]\left(f-g\right)\left(x\right)[/latex].
Are they the same function?
[latex]\left(fg\right)\left(x\right)=f\left(x\right)\cdot g\left(x\right)=\left(x - 1\right)\left({x}^{2}-1\right)={x}^{3}-{x}^{2}-x+1\\[4mm] \left(f-g\right)\left(x\right)=f\left(x\right)-g\left(x\right)=\left(x - 1\right)-\left({x}^{2}-1\right)=x-{x}^{2}[/latex]
Create a Function by Composition of Functions
Performing algebraic operations on functions combines them into a new function, but we can also create functions by composing functions. When we wanted to compute a heating cost from a day of the year, we created a new function that takes a day as input and yields a cost as output. The process of combining functions so that the output of one function becomes the input of another is known as a composition of functions. The resulting function is known as a composite function. We represent this combination by the following notation:
[latex]\left(f\circ g\right)\left(x\right)=f\left(g\left(x\right)\right)[/latex]
We read the left-hand side as [latex]``f[/latex] composed with [latex]g[/latex] at [latex]x,''[/latex] and the right-hand side as [latex]``f[/latex] of [latex]g[/latex] of [latex]x.''[/latex] The two sides of the equation have the same mathematical meaning and are equal. The open circle symbol [latex]\circ [/latex] is called the composition operator. We use this operator mainly when we wish to emphasize the relationship between the functions themselves without referring to any particular input value. Composition is a binary operation that takes two functions and forms a new function, much as addition or multiplication takes two numbers and gives a new number. However, it is important not to confuse function composition with multiplication because, as we learned above, in most cases [latex]f\left(g\left(x\right)\right)\ne f\left(x\right)g\left(x\right)[/latex].
It is also important to understand the order of operations in evaluating a composite function. We follow the usual convention with parentheses by starting with the innermost parentheses first, and then working to the outside. In the equation above, the function [latex]g[/latex] takes the input [latex]x[/latex] first and yields an output [latex]g\left(x\right)[/latex]. Then the function [latex]f[/latex] takes [latex]g\left(x\right)[/latex] as an input and yields an output [latex]f\left(g\left(x\right)\right)[/latex].

In general, [latex]f\circ g[/latex] and [latex]g\circ f[/latex] are different functions. In other words, in many cases [latex]f\left(g\left(x\right)\right)\ne g\left(f\left(x\right)\right)[/latex] for all [latex]x[/latex]. We will also see that sometimes two functions can be composed only in one specific order.
For example, if [latex]f\left(x\right)={x}^{2}[/latex] and [latex]g\left(x\right)=x+2[/latex], then
[latex]\begin{align}f\left(g\left(x\right)\right)&=f\left(x+2\right) \\ &={\left(x+2\right)}^{2} \\ &={x}^{2}+4x+4 \end{align}[/latex]
[latex]\begin{align} g\left(f\left(x\right)\right)&=g\left({x}^{2}\right)\\ &={x}^{2}+2 \end{align}[/latex]
These expressions are not equal for all values of [latex]x[/latex], so the two functions are not equal.
Note that the range of the inside function (the first function to be evaluated) needs to be within the domain of the outside function. Less formally, the composition has to make sense in terms of inputs and outputs.
A General Note: Composition of Functions
When the output of one function is used as the input of another, we call the entire operation a composition of functions. For any input [latex]x[/latex] and functions [latex]f[/latex] and [latex]g[/latex], this action defines a composite function, which we write as [latex]f\circ g[/latex] such that
The domain of the composite function [latex]f\circ g[/latex] is all [latex]x[/latex] such that [latex]x[/latex] is in the domain of [latex]g[/latex] and [latex]g\left(x\right)[/latex] is in the domain of [latex]f[/latex].
It is important to realize that the product of functions [latex]fg[/latex] is not the same as the function composition [latex]f\left(g\left(x\right)\right)[/latex], because, in general, [latex]f\left(x\right)g\left(x\right)\ne f\left(g\left(x\right)\right)[/latex].
Example 2: Determining whether Composition of Functions is Commutative
Using the functions below, find [latex]f\left(g\left(x\right)\right)[/latex] and [latex]g\left(f\left(x\right)\right)[/latex]. Determine whether the composition of the functions is commutative.
[latex]f\left(x\right)=2x+1[/latex] [latex]g\left(x\right)=3-x[/latex]
Let’s begin by substituting [latex]g\left(x\right)[/latex] into [latex]f\left(x\right)[/latex].
[latex]\begin{align}f\left(g\left(x\right)\right)&=2\left(3-x\right)+1 \\ &=6 - 2x+1 \\ &=7 - 2x \end{align}[/latex]
Now we can substitute [latex]f\left(x\right)[/latex] into [latex]g\left(x\right)[/latex].
[latex]\begin{align}g\left(f\left(x\right)\right)&=3-\left(2x+1\right) \\ &=3 - 2x - 1 \\ &=-2x+2 \end{align}[/latex]
We find that [latex]g\left(f\left(x\right)\right)\ne f\left(g\left(x\right)\right)[/latex], so the operation of function composition is not commutative.
Example 3: Interpreting Composite Functions
The function [latex]c\left(s\right)[/latex] gives the number of calories burned completing [latex]s[/latex] sit-ups, and [latex]s\left(t\right)[/latex] gives the number of sit-ups a person can complete in [latex]t[/latex] minutes. Interpret [latex]c\left(s\left(3\right)\right)[/latex].
The inside expression in the composition is [latex]s\left(3\right)[/latex]. Because the input to the s-function is time, [latex]t=3[/latex] represents 3 minutes, and [latex]s\left(3\right)[/latex] is the number of sit-ups completed in 3 minutes.
Using [latex]s\left(3\right)[/latex] as the input to the function [latex]c\left(s\right)[/latex] gives us the number of calories burned during the number of sit-ups that can be completed in 3 minutes, or simply the number of calories burned in 3 minutes (by doing sit-ups).
Example 4: Investigating the Order of Function Composition
Suppose [latex]f\left(x\right)[/latex] gives miles that can be driven in [latex]x[/latex] hours and [latex]g\left(y\right)[/latex] gives the gallons of gas used in driving [latex]y[/latex] miles. Which of these expressions is meaningful: [latex]f\left(g\left(y\right)\right)[/latex] or [latex]g\left(f\left(x\right)\right)?[/latex]
The function [latex]y=f\left(x\right)[/latex] is a function whose output is the number of miles driven corresponding to the number of hours driven.
[latex]\text{number of miles }=f\left(\text{number of hours}\right)[/latex]
The function [latex]g\left(y\right)[/latex] is a function whose output is the number of gallons used corresponding to the number of miles driven. This means:
[latex]\text{number of gallons }=g\left(\text{number of miles}\right)[/latex]
The expression [latex]g\left(y\right)[/latex] takes miles as the input and a number of gallons as the output. The function [latex]f\left(x\right)[/latex] requires a number of hours as the input. Trying to input a number of gallons does not make sense. The expression [latex]f\left(g\left(y\right)\right)[/latex] is meaningless.
The expression [latex]f\left(x\right)[/latex] takes hours as input and a number of miles driven as the output. The function [latex]g\left(y\right)[/latex] requires a number of miles as the input. Using [latex]f\left(x\right)[/latex] (miles driven) as an input value for [latex]g\left(y\right)[/latex], where gallons of gas depends on miles driven, does make sense. The expression [latex]g\left(f\left(x\right)\right)[/latex] makes sense, and will yield the number of gallons of gas used, [latex]g[/latex], driving a certain number of miles, [latex]f\left(x\right)[/latex], in [latex]x[/latex] hours.
Are there any situations where [latex]f\left(g\left(y\right)\right)[/latex] and [latex]g\left(f\left(x\right)\right)[/latex] would both be meaningful or useful expressions?
Yes. For many pure mathematical functions, both compositions make sense, even though they usually produce different new functions. In real-world problems, functions whose inputs and outputs have the same units also may give compositions that are meaningful in either order.
The gravitational force on a planet a distance r from the sun is given by the function [latex]G\left(r\right)[/latex]. The acceleration of a planet subjected to any force [latex]F[/latex] is given by the function [latex]a\left(F\right)[/latex]. Form a meaningful composition of these two functions, and explain what it means.
A gravitational force is still a force, so [latex]a\left(G\left(r\right)\right)[/latex] makes sense as the acceleration of a planet at a distance r from the Sun (due to gravity), but [latex]G\left(a\left(F\right)\right)[/latex] does not make sense.
Evaluating Composite Functions
Once we compose a new function from two existing functions, we need to be able to evaluate it for any input in its domain. We will do this with specific numerical inputs for functions expressed as tables, graphs, and formulas and with variables as inputs to functions expressed as formulas. In each case, we evaluate the inner function using the starting input and then use the inner function’s output as the input for the outer function.
Evaluating Composite Functions Using Tables
When working with functions given as tables, we read input and output values from the table entries and always work from the inside to the outside. We evaluate the inside function first and then use the output of the inside function as the input to the outside function.
Example 5: Using a Table to Evaluate a Composite Function
Using the table below, evaluate [latex]f\left(g\left(3\right)\right)[/latex] and [latex]g\left(f\left(3\right)\right)[/latex].
| [latex]x[/latex] | [latex]f\left(x\right)[/latex] | [latex]g\left(x\right)[/latex] |
|---|---|---|
| 1 | 6 | 3 |
| 2 | 8 | 5 |
| 3 | 3 | 2 |
| 4 | 1 | 7 |
To evaluate [latex]f\left(g\left(3\right)\right)[/latex], we start from the inside with the input value 3. We then evaluate the inside expression [latex]g\left(3\right)[/latex] using the table that defines the function [latex]g:[/latex] [latex]g\left(3\right)=2[/latex]. We can then use that result as the input to the function [latex]f[/latex], so [latex]g\left(3\right)[/latex] is replaced by 2 and we get [latex]f\left(2\right)[/latex]. Then, using the table that defines the function [latex]f[/latex], we find that [latex]f\left(2\right)=8[/latex].
[latex]g\left(3\right)=2[/latex]
[latex]f\left(g\left(3\right)\right)=f\left(2\right)=8[/latex]
To evaluate [latex]g\left(f\left(3\right)\right)[/latex], we first evaluate the inside expression [latex]f\left(3\right)[/latex] using the first table: [latex]f\left(3\right)=3[/latex]. Then, using the table for [latex]g[/latex] we can evaluate
[latex]g\left(f\left(3\right)\right)=g\left(3\right)=2[/latex]
The table below shows the composite functions [latex]f\circ g[/latex] and [latex]g\circ f[/latex] as tables.
| [latex]x[/latex] | [latex]g\left(x\right)[/latex] | [latex]f\left(g\left(x\right)\right)[/latex] | [latex]f\left(x\right)[/latex] | [latex]g\left(f\left(x\right)\right)[/latex] |
| 3 | 2 | 8 | 3 | 2 |
Using the table below, evaluate [latex]f\left(g\left(1\right)\right)[/latex] and [latex]g\left(f\left(4\right)\right)[/latex].
[latex]f\left(g\left(1\right)\right)=f\left(3\right)=3[/latex] and [latex]g\left(f\left(4\right)\right)=g\left(1\right)=3[/latex]
Evaluating Composite Functions Using Graphs
When we are given individual functions as graphs, the procedure for evaluating composite functions is similar to the process we use for evaluating tables. We read the input and output values, but this time, from the [latex]x\text{-}[/latex] and [latex]y\text{-}[/latex] axes of the graphs.
How To: Given a composite function and graphs of its individual functions, evaluate it using the information provided by the graphs.
- Locate the given input to the inner function on the [latex]x\text{-}[/latex] axis of its graph.
- Read off the output of the inner function from the [latex]y\text{-}[/latex] axis of its graph.
- Locate the inner function output on the [latex]x\text{-}[/latex] axis of the graph of the outer function.
- Read the output of the outer function from the [latex]y\text{-}[/latex] axis of its graph. This is the output of the composite function.
Example 6: Using a Graph to Evaluate a Composite Function
Using the graphs in Figure 3, evaluate [latex]f\left(g\left(1\right)\right)[/latex].
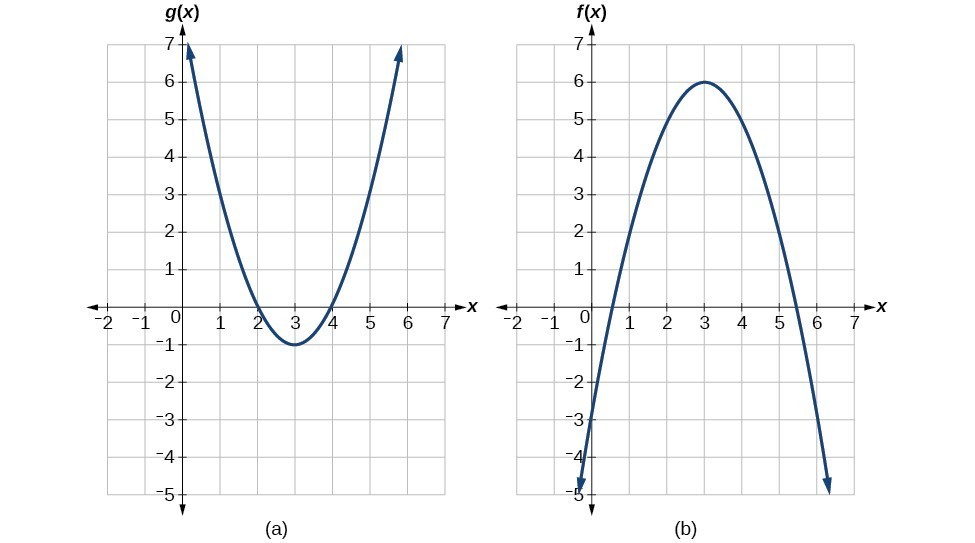
To evaluate [latex]f\left(g\left(1\right)\right)[/latex], we start with the inside evaluation.
We evaluate [latex]g\left(1\right)[/latex] using the graph of [latex]g\left(x\right)[/latex], finding the input of 1 on the [latex]x\text{-}[/latex] axis and finding the output value of the graph at that input. Here, [latex]g\left(1\right)=3[/latex]. We use this value as the input to the function [latex]f[/latex].
[latex]f\left(g\left(1\right)\right)=f\left(3\right)[/latex]
We can then evaluate the composite function by looking to the graph of [latex]f\left(x\right)[/latex], finding the input of 3 on the [latex]x\text{-}[/latex] axis and reading the output value of the graph at this input. Here, [latex]f\left(3\right)=6[/latex], so [latex]f\left(g\left(1\right)\right)=6[/latex].
Figure 5 shows how we can mark the graphs with arrows to trace the path from the input value to the output value.

Using Figure 6, evaluate [latex]g\left(f\left(2\right)\right)[/latex].
[latex]g\left(f\left(2\right)\right)=g\left(5\right)=3[/latex]
Evaluating Composite Functions Using Formulas
When evaluating a composite function where we have either created or been given formulas, the rule of working from the inside out remains the same. The input value to the outer function will be the output of the inner function, which may be a numerical value, a variable name, or a more complicated expression.
While we can compose the functions for each individual input value, it is sometimes helpful to find a single formula that will calculate the result of a composition [latex]f\left(g\left(x\right)\right)[/latex]. To do this, we will extend our idea of function evaluation. Recall that, when we evaluate a function like [latex]f\left(t\right)={t}^{2}-t[/latex], we substitute the value inside the parentheses into the formula wherever we see the input variable.
How To: Given a formula for a composite function, evaluate the function.
- Evaluate the inside function using the input value or variable provided.
- Use the resulting output as the input to the outside function.
Example 7: Evaluating a Composition of Functions Expressed as Formulas with a Numerical Input
Given [latex]f\left(t\right)={t}^{2}-{t}[/latex] and [latex]h\left(x\right)=3x+2[/latex], evaluate [latex]f\left(h\left(1\right)\right)[/latex].
Because the inside expression is [latex]h\left(1\right)[/latex], we start by evaluating [latex]h\left(x\right)[/latex] at 1.
[latex]\begin{align}h\left(1\right)&=3\left(1\right)+2\\ &=5\end{align}[/latex]
Then [latex]f\left(h\left(1\right)\right)=f\left(5\right)[/latex], so we evaluate [latex]f\left(t\right)[/latex] at an input of 5.
[latex]\begin{align}f\left(h\left(1\right)\right)&=f\left(5\right)\\ &={5}^{2}-5\\ &=20\end{align}[/latex]
It makes no difference what the input variables [latex]t[/latex] and [latex]x[/latex] were called in this problem because we evaluated for specific numerical values.
Given [latex]f\left(t\right)={t}^{2}-t[/latex] and [latex]h\left(x\right)=3x+2[/latex], evaluate
A) [latex]h\left(f\left(2\right)\right)[/latex]
B) [latex]h\left(f\left(-2\right)\right)[/latex]
A. 8; B. 20
Finding the Domain of a Composite Function
As we discussed previously, the domain of a composite function such as [latex]f\circ g[/latex] is dependent on the domain of [latex]g[/latex] and the domain of [latex]f[/latex]. It is important to know when we can apply a composite function and when we cannot, that is, to know the domain of a function such as [latex]f\circ g[/latex]. Let us assume we know the domains of the functions [latex]f[/latex] and [latex]g[/latex] separately. If we write the composite function for an input [latex]x[/latex] as [latex]f\left(g\left(x\right)\right)[/latex], we can see right away that [latex]x[/latex] must be a member of the domain of [latex]g[/latex] in order for the expression to be meaningful, because otherwise we cannot complete the inner function evaluation. However, we also see that [latex]g\left(x\right)[/latex] must be a member of the domain of [latex]f[/latex], otherwise the second function evaluation in [latex]f\left(g\left(x\right)\right)[/latex] cannot be completed, and the expression is still undefined. Thus the domain of [latex]f\circ g[/latex] consists of only those inputs in the domain of [latex]g[/latex] that produce outputs from [latex]g[/latex] belonging to the domain of [latex]f[/latex]. Note that the domain of [latex]f[/latex] composed with [latex]g[/latex] is the set of all [latex]x[/latex] such that [latex]x[/latex] is in the domain of [latex]g[/latex] and [latex]g\left(x\right)[/latex] is in the domain of [latex]f[/latex].
A General Note: Domain of a Composite Function
The domain of a composite function [latex]f\left(g\left(x\right)\right)[/latex] is the set of those inputs [latex]x[/latex] in the domain of [latex]g[/latex] for which [latex]g\left(x\right)[/latex] is in the domain of [latex]f[/latex].
How To: Given a function composition [latex]f\left(g\left(x\right)\right)[/latex], determine its domain.
- Find the domain of [latex]g[/latex].
- Find the domain of [latex]f[/latex].
- Find those inputs, [latex]x[/latex], in the domain of [latex]g[/latex] for which [latex]g(x)[/latex] is in the domain of [latex]f[/latex]. That is, exclude those inputs, [latex]x[/latex], from the domain of [latex]g[/latex] for which [latex]g(x)[/latex] is not in the domain of [latex]f[/latex]. The resulting set is the domain of [latex]f\circ g[/latex].
Example 8: Finding the Domain of a Composite Function
Find the domain of
[latex]\left(f\circ g\right)\left(x\right)\text{ where}f\left(x\right)=\frac{5}{x - 1}\text{ and }g\left(x\right)=\frac{4}{3x - 2}[/latex]
The domain of [latex]g\left(x\right)[/latex] consists of all real numbers except [latex]x=\frac{2}{3}[/latex], since that input value would cause us to divide by 0. Likewise, the domain of [latex]f[/latex] consists of all real numbers except 1. So we need to exclude from the domain of [latex]g\left(x\right)[/latex] that value of [latex]x[/latex] for which [latex]g\left(x\right)=1[/latex].
[latex]\begin{align}\frac{4}{3x - 2}&=1 \\ 4&=3x - 2 &&\text{Multiply by } 3x-2 \\ 6&=3x &&\text{Add }2 \\ x&=2 && \text{Divide by }3 \end{align}[/latex]
So the domain of [latex]f\circ g[/latex] is the set of all real numbers except [latex]\frac{2}{3}[/latex] and [latex]2[/latex]. This means that
[latex]x\ne \frac{2}{3}\text{or}x\ne 2[/latex]
We can write this in interval notation as
[latex]\left(-\infty ,\frac{2}{3}\right)\cup \left(\frac{2}{3},2\right)\cup \left(2,\infty \right)[/latex]
Example 9: Finding the Domain of a Composite Function Involving Radicals
[latex]\left(f\circ g\right)\left(x\right)\text{ where}f\left(x\right)=\sqrt{x+2}\text{ and }g\left(x\right)=\sqrt{3-x}[/latex]
Because we cannot take the square root of a negative number, the domain of [latex]g[/latex] is [latex]\left(-\infty ,3\right][/latex]. Now we check the domain of the composite function
[latex]\left(f\circ g\right)\left(x\right)=\sqrt{3-x+2}\text{ or }\left(f\circ g\right)\left(x\right)=\sqrt{5-x}[/latex]
The domain of this function is [latex]\left(-\infty ,5\right][/latex]. To find the domain of [latex]f\circ g[/latex], we ask ourselves if there are any further restrictions offered by the domain of the composite function. The answer is no, since [latex]\left(-\infty ,3\right][/latex] is a proper subset of the domain of [latex]f\circ g[/latex]. This means the domain of [latex]f\circ g[/latex] is the same as the domain of [latex]g[/latex], namely, [latex]\left(-\infty ,3\right][/latex].
This example shows that knowledge of the range of functions (specifically the inner function) can also be helpful in finding the domain of a composite function. It also shows that the domain of [latex]f\circ g[/latex] can contain values that are not in the domain of [latex]f[/latex], though they must be in the domain of [latex]g[/latex].
[latex]\left(f\circ g\right)\left(x\right)\text{ where}f\left(x\right)=\frac{1}{x - 2}\text{ and }g\left(x\right)=\sqrt{x+4}[/latex]
[latex]\left[-4,0\right)\cup \left(0,\infty \right)[/latex]
Decomposing a Composite Function into its Component Functions
In some cases it is necessary to decompose a complicated function. In other words we can write it as a composition of two simpler functions. There are multiple ways to decompose a composite function , but often one is the most obvious.
Example 10: Decomposing a Function
Write [latex]f\left(x\right)=\sqrt{5-{x}^{2}}[/latex] as the composition of two functions.
We are looking for two functions, [latex]g[/latex] and [latex]h[/latex], so [latex]f\left(x\right)=g\left(h\left(x\right)\right)[/latex]. To do this, we look for a function inside a function in the formula for [latex]f\left(x\right)[/latex]. As one possibility, we might notice that the expression [latex]5-{x}^{2}[/latex] is the inside of the square root. We could then decompose the function as
[latex]h\left(x\right)=5-{x}^{2}\text{ and }g\left(x\right)=\sqrt{x}[/latex]
We can check our answer by recomposing the functions.
[latex]g\left(h\left(x\right)\right)=g\left(5-{x}^{2}\right)=\sqrt{5-{x}^{2}}[/latex]
Another solution would be
[latex]h\left(x\right)={x}^{2}\text{ and }g\left(x\right)=\sqrt{5-x}[/latex]
Write [latex]f\left(x\right)=\frac{4}{3-\sqrt{4+{x}^{2}}}[/latex] as the composition of two functions.
Possible answer:
[latex]g\left(x\right)=\sqrt{4+{x}^{2}}[/latex]
[latex]h\left(x\right)=\frac{4}{3-x}[/latex]
[latex]f=h\circ g[/latex]
Key Equations
| Composite function | [latex]\left(f\circ g\right)\left(x\right)=f\left(g\left(x\right)\right)[/latex] |
| Average rate of change | [latex]\dfrac{\Delta y}{\Delta x}=\dfrac{f\left({x}_{2}\right)-f\left({x}_{1}\right)}{{x}_{2}-{x}_{1}}[/latex] |
Key Concepts
- A rate of change relates a change in an output quantity to a change in an input quantity. The average rate of change is determined using only the beginning and ending data.
- Identifying points that mark the interval on a graph can be used to find the average rate of change.
- Comparing pairs of input and output values in a table can also be used to find the average rate of change.
- An average rate of change can also be computed by determining the function values at the endpoints of an interval described by a formula.
- The average rate of change can sometimes be determined as an expression.
- A function is increasing where its rate of change is positive and decreasing where its rate of change is negative.
- A local maximum is where a function changes from increasing to decreasing and has an output value larger (more positive or less negative) than output values at neighboring input values.
- A local minimum is where the function changes from decreasing to increasing (as the input increases) and has an output value smaller (more negative or less positive) than output values at neighboring input values.
- Minima and maxima are also called extrema.
- We can find local extrema from a graph.
- When functions are combined, the output of the first (inner) function becomes the input of the second (outer) function.
- The function produced by combining two functions is a composite function.
- The order of function composition must be considered when interpreting the meaning of composite functions.
- A composite function can be evaluated by evaluating the inner function using the given input value and then evaluating the outer function taking as its input the output of the inner function.
- A composite function can be evaluated from a table.
- A composite function can be evaluated from a graph.
- A composite function can be evaluated from a formula.
- The domain of a composite function consists of those inputs in the domain of the inner function that correspond to outputs of the inner function that are in the domain of the outer function.
- Just as functions can be combined to form a composite function, composite functions can be decomposed into simpler functions.
- Functions can often be decomposed in more than one way.
- http://www.eia.gov/totalenergy/data/annual/showtext.cfm?t=ptb0524 . Accessed 3/5/2014. ↵
- Precalculus. Authored by : OpenStax College. Provided by : OpenStax. Located at : http://cnx.org/contents/[email protected]:1/Preface . License : CC BY: Attribution

- Burger Junior High School
- Grade 8 Math
- Class Schedule
Falci, Jakob
Page navigation.
- Mr. Falci's Home Page
- Unit A1 Key Vocabulary Flash Cards
- Class Notes and Online Resources
- Extra Practice Worksheets with Answers
- Video Tutorials
- Video Tutorials - Identity and Inverse Properties
- Video Tutorials - Commutative and Associative Properties
- Video Tutorials - Greatest Common Factor
- Video Tutorials - Least Common Multiple
- Video Tutorials - Prime Factorization
- Unit A1 Retesting Resources
- Unit A2 Key Vocabulary Flash Cards
- Unit A2 Retesting Resources
- Pre-Unit Study Materials
- Unit B Key Vocabulary Flash Cards
- Video Tutorials and Class Notes
- Unit B Retesting Resources
- Pre-Unit Learning Resources
- Unit C Key Vocabulary Flash Cards
- Unit C Retesting Resources
- Unit D Key Vocabulary Flash Cards
- Unit D Retesting Resources
- Unit E Key Vocabulary Flash Cards
- Unit E Retesting Page
- Unit F Key Vocabulary Flash Cards
- Beginning of the Year Skills Stations
- Writing Expressions, Equations and Inequilities Stations
- Equivalent Expressions and GCF/LCM
- Operations with Fractions, Decimals, and Solving Equations
- Graphing, Rationals, Rates, and Volume Review Stations
- Percents and Area Stations
- Unit Review Flash Cards
- Unit Test Retesting
- Weekly Reviews
- Unit 1: Volume
- Unit 2: Equations
Unit 3: Introduction to Functions
- Unit 4: Linear Functions
- Unit 5: Systems of Linear Equations
- Unit 6: Exponent Rules
- Unit 8: Congruence
- Unit 9: Similarity
- Unit 10: Angle Relationships
- Unit 1: Equations
- Unit 2: Inequalities
- Unit 6: Polynomials and Factoring
- Unit 7: Key Features of Quadratics
- Unit 8: Solving Quadratic Equations
- Unit 9: Exponential Functions
- Weekly Reviews/Problem of the Week
- Solving Equations
- Solving Inequalities
- Exponents and Exponential Functions
- Polynomials and Factoring
- Graphs and Functions
- Linear Equations and Their Graphs
- Quadratic Equations and Functions
- Systems of Equations
- Radicals and Trigonometry
- Rational Expressions and Functions
- Algebra Regents Exam ANSWERS
- Variables, Functions, and Graphs
- Properties and Probability
- Unit 1 - Transformations
- Unit 2 - Tools of Geometry
- Unit 3 - Reasoning and Proof
- Unit 4 - Parallel and Perpendicular Lines
- Unit 5 - Triangle Relationships
- Unit 6 - Congruent Triangles
- Unit 7 - Quadrilaterals
- Unit 8 - Similarity
- Unit 9 - Right Triangles and Area of Polygons
- Unit 10 - Volume and Surface Area
- Unit 11 - Circles
- Unit 12 - Equation of Circle, Locus and Constructions
- Construction Tutorials
- Geometry Regents Review
- Geometry Regents Exam ANSWERS
- Extra Practice for RETESTING
- Review Sheet ANSWERS
Friday, November 4
- Homework: END OF MARKING PERIOD - TUESDAY 11/8
Day 14: Intro to Functions TEST Day 1
Thursday, November 3
- Intro to Functions Unit Test
- Thursday 11/3 and Friday 11/4
- Homework: Intro to Functions Unit Review - Due 11/4
Day 13: Intro to Functions Review Day 2
Wednesday, November 2
- Class Work: Intro to Functions Review Stations
Day 12: Intro to Functions Review Day 1
Tuesday, November 1
- Warm-Up: Function Notation
- Class Work: Intro to Functions Graded Assignment
Day 11: Linear Functions In the Real-World
Monday, October 31
- Class Work: Rate of Change from Tables and Graphs
- Class Notes: Linear Functions Day 2
Day 10: Linear Functions - Rate of Change
Friday, October 28
- Class Work: Graphing Functions Warm-Up
- Class Notes: Linear Functions Day 1
- Homework: None
Day 9: Function Tables and Graphs Day 2
Thursday, October 27
- Class Notes: Function Tables and Graphs - Exponential and Absolute Value
Day 8: Function Tables and Graphs Day 1
Wednesday, October 26
- Class Notes: Function Tables and Graphs - Linear and Quadratic
- Homework: Practice 5-3
Day 7: Function Notations
Tuesday, October 25
- Class Notes: Function Notation
Day 6: Functions: Vertical Line Test
Monday, October 24
- Class Work: Mapping Diagrams
- Class Notes: Relations and Functions (Vertical Line Test)
- Homework: Practice 5-2b
Day 5: Functions: Mapping Diagrams
Friday, October 21
- Class Work: Function Tables and Function Rules
- Class Notes: Relations and Functions (Mapping Diagrams)
Day 4: Continuous and Discrete Data
Thursday, October 20
- Class Notes: Patterns and Functions
Day 3: Patterns and Functions
Wednesday, October 19
- Homework: Practice 5-2a Worksheet
Tuesday, October 18
- Class Work: Graphing Stories Launch
- Homework: Practice 5-1 Worksheet
Monday, October 17
- Class Work: Relating Graphs to Events
- Questions or Feedback? |
- Web Community Manager Privacy Policy (Updated) |

IMAGES
VIDEO
COMMENTS
Lesson 3-5 Graphs of Relations and Functions. Relation and function revisited. Discrete and Continuous Data. Determining the domain and range of a function / relation. Expressing the domain and range of a function / relation. Assignment Key. U2S1C5 - Part 4. Lesson 3-6 Properties of Linear Relations. Linear and Non-Linear Relations.
Range: -3, 7, 10. Draw the mapping diagram for the relation and determine whether it is a function or not. { (2, 4), (- 8, 0), (1, 5), (3, 1)} Yes, it is a function. Study with Quizlet and memorize flashcards containing terms like Express the relation as a table and a graph.
Example 3.2.21. For the function f(x) = 2x2 + 4x − 3, evaluate the function. f(2) f( − 3) f(h) Answer. In the last example, we found f(x) for a constant value of x. In the next example, we are asked to find g(x) with values of x that are variables. We still follow the same procedure and substitute the variables in for the x.
This Relations and Functions Unit Bundle contains guided notes, homework assignments, two quizzes, a study guide, and a unit test that cover the following topics: • Coordinate Plane and Graphing Review. • Relations. • Representing Relations (Tables, Graphs, Ordered Pairs, Mappings) • Domain and Range of Ordered Pairs.
GRAPH A GRAPH B 9. Frank flew from Japan to Australia. Which graph best represents his distance to the Equator during the flight? GRAPH A GRAPH B 10. Kiara walked up a hill at a steady pace, then ran down the hill. Which graph best represents her speed? GRAPH A GRAPH B 11. Describe a real-life situation that would create the graph to the right.
(cK-12 Flexbook Unit 3 Topic 1 SLT 8) • Use the sine and cosine functions to model real-world phenomena. (cK-12 Flexbook Unit 3 Topic 1 SLT 9) • Define and graph the tangent function and identify key features of the graph. (cK-12 Flexbook Unit 3 Topic 1 SLT 10) • Make connections among a variety of function types, their ...
Free worksheet(pdf) and answer key on distinguishing functions from relations, stating domain and range and more
These relations and functions guided notes and worksheets cover:relation and function vocabularyways to represent relationshow to determine if a relation is a functioncoordinate plane reviewnotating and evaluating functionsanalyzing graphs (domain, range, continuous, discrete, zeros, intervals of increase and decrease)real-world graphs16 pages + all answer keys includedYou may also like ...
4.9. (26) $3.50. PDF. Systems of Equations and Inequalities - Real World Applications: this handout challenges students to apply their algebra skills in real world situations. The applications can be used as a way to measure student growth or for review. Topics included are: • Systems of equations and inequalities: Linear to Linear and Linear ...
Get a hint. Constant function. f (x)=b,b is a real number. Click the card to flip 👆. special linear ( m=0) domain and range; all real numbers. horizontal graph with y-intercept is b. Click the card to flip 👆. 1 / 9.
Use the Fundamental Theorem of Algebra to determine the number of zeros of polynomial functions. pg 308 #1-6. Find rational zeros of polynomial functions. pg 308 #11-20. Find conjugate pairs of complex zeros. pg 309 #47-54. Find zeros of polynomials by factoring. pg 309 #55-72. Write the equations of polynomials functions given the zeros and ...
Math 108X Lesson 8 Functions and Their Graphs; Math 108x Lesson 4 Tools for Building Better Budgets and More; Math for the Real World Lesson 3 Logical Reasoning; FDMAT108 Week #6 NOtes; Fdmat Week #7 Notes; ... Math For The Real World (FDMAT108) 2 months ago. Juan is a competitive swimmer. He would like to optimize his post-workout meal, so he ...
Day Math Learning Goal Homework 1 Getting Started: A review of linear and algebraic concepts p. 130 #1-10 2 3.1: Exploring Quadratic Relations Activity p. 136 # 1-3, 4ace, 5-7 3 3.2: Properties of Graphs of Quadratic Relations p. 146 # 4-6, 7ace, 9ac, 10 4 Using the Graphing Calculators to Model Quadratics in the Real World
Algebra 2 -25 - Functions, Equations, and Graphs WARM UP Solve each equation for y. 1) 12y=3x 2) −10y=5x 3) 3 4 y=15x y= 1 4 x y=− 1 2 x y=20x KEY CONCEPTS AND VOCABULARY Direct Variation- a linear function defined by an equation of the form y=kx, where k ≠ 0. Constant of Variation - k, where k = y/x GRAPHS OF DIRECT VARIATIONS
Homework 2 Real World Graphs Worksheets - total of 8 printable worksheets available for this concept. Worksheets are Systems of equations real world g...
Khanmigo is now free for all US educators! Plan lessons, develop exit tickets, and so much more with our AI teaching assistant.
Explore math with our beautiful, free online graphing calculator. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more.
If we were interested only in how the gasoline prices changed between 2005 and 2012, we could compute that the cost per gallon had increased from $2.31 to $3.68, an increase of $1.37.
Unit 3: Introduction to Functions. Below is the Current Schedule for Unit 3: Introduction to Functions with Notes, Homework, and any Class Activities that we did on the given dates. Day 15: Intro to Functions TEST Day 2. Friday, November 4. Homework: END OF MARKING PERIOD - TUESDAY 11/8.
Unit 2- Functions and their graphs. Flashcards; Learn; Test; Match; Q-Chat; Get a hint. Polynomial End Behavior ... Part of Sound-Stoudt chapter 3. 11 terms. avawerner99. Preview. HY Western Civilization study guide 3. 30 terms. JDWZ. Preview. Macro Final exam part 1. 21 terms. way_matt3457. Preview. Unit 2 Real Property and the Law. Teacher 21 ...
Our expert help has broken down your problem into an easy-to-learn solution you can count on. Question: Name: Date: Unit 3: Relations and Functions Per: Homework 1: Relations, Domain Range and Functions is a 2-poge document Idently the domain and range, then represent the relation as a table, mapping, and graph. 1.